Medians and centroid
A median of a triangle is a line that passes through a vertex and the midpoint of the opposite side. The three medians of a triangle intersect at one and the same point, which is called the centroid of the triangle.
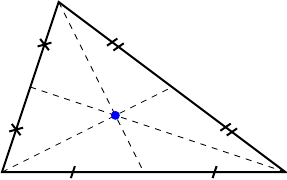
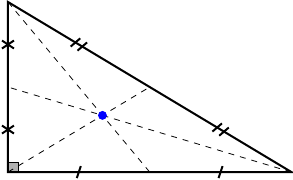
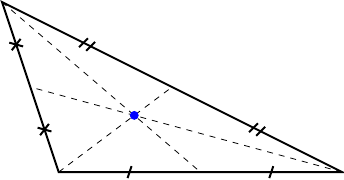
Theorems of medians and centroid
- A median divides the triangle into two triangles of equal area
- The centroid of a triangle is the centre of mass of the triangle.
- The centroid divides a median in 2:1 ratio from the vertex to the base.
- The segment that connects two medians is parallel to the third side of the triangle.